CAT 2018 Question Paper | CAT LR DI

CAT Questions

Here is an exacting problem from the CAT 2018 exam. You are required to find the 'Bravo's' revenue according to the given constraints. This puzzle turned out to be a nightmare for the inexperienced puzzle solvers since it presents the data in a slightly unorthodox way. It is highly recommended to solve a gazillion questions from the CAT question bank to get an idea about the advanced-level CAT puzzles. Now solve this problem and ace your Online CAT Preparation

CAT DILR : CAT 2018 Question Paper Slot 2

Set 3 : Fun Sports Club

Fun Sports (FS) provides training in three sports - Gilli-danda (G), Kho-Kho (K), and Ludo (L). Currently it has an enrollment of 39 students each of whom is enrolled in at least one of the three sports. The following details are known:
1. The number of students enrolled only in L is double the number of students enrolled in all the three sports.
2. There are a total of 17 students enrolled in G.
3. The number of students enrolled only in G is one less than the number of students enrolled only in L.
4. The number of students enrolled only in K is equal to the number of students who are enrolled in both K and L.
5. The maximum student enrollment is in L.
6. Ten students enrolled in G are also enrolled in at least one more sport.

Question 4 : Due to academic pressure, students who were enrolled in all three sports were asked to withdraw from one of the three sports. After the withdrawal, the number of students enrolled in G was six less than the number of students enrolled in L, while the number of students enrolled in K went down by one.After the withdrawal, how many students were enrolled in both G and L?

1. 5
2. 8
3. 7
4. 6

---

---

Video Explanation

---

---

Explanatory Answer

Method of solving this CAT Question on CAT DILR

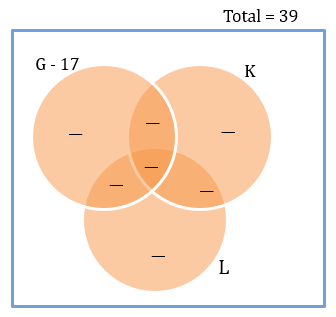
From condition 3, we get the above diagram.

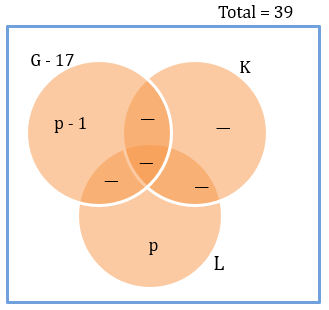
Condition 6 tells us p – 1 = 7. G-total = 17. G and something else = 10. G-only should be 7.

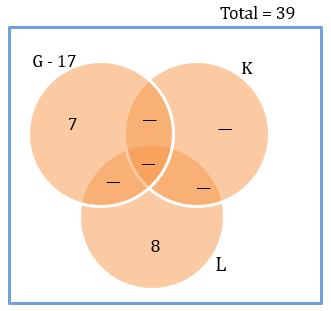
From condition 1, we get the above diagram.

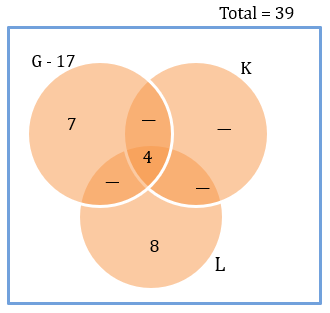
From condition 6, we can get the diagram.

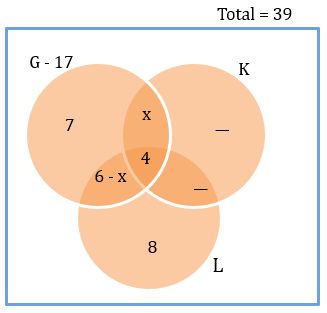
From condition 4, we get the above diagram.

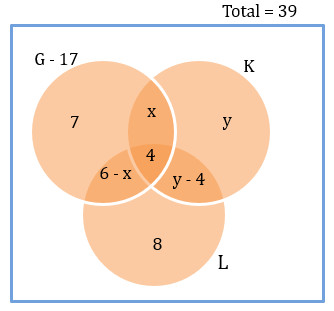
Now we know that the total = 39.

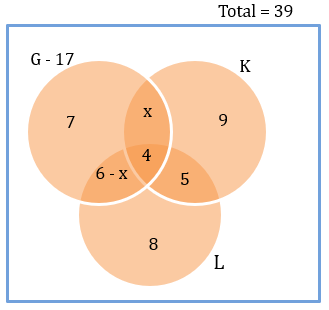
From condition 5,
Number of students in L = 4 + 5 + 8 + 6 – x = 23- x.
Number of students in K = 4 + 5 + 9 + x = 18 + x.
We know that 23 – x > 18 + x.
5 > 2x. x < \\frac{5}{2}).
x could be 0, 1 or 2.


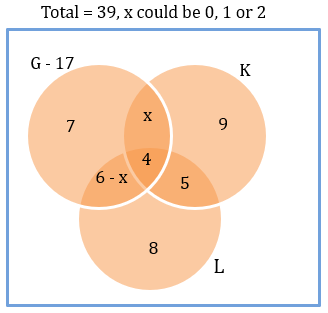
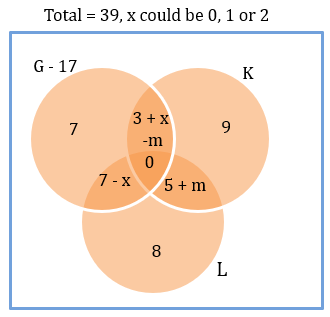
The other three should have opted out of one or the other of G and L. Let us assume m students left G, 3 – m should have left L.
Let us rejig the diagram.
Total number of students in G = 17 – m.
Total number of students in L = 20 + m – x.
20 + m – x – (17 – m) = 3 + 2m – x = 6.
2m – x = 3. x can only take values 0, 1 and 2. 2m = 3 + x.
Or, x has to be 1. m has to be 2.
Both G and L = 7 – x = 6.

The question is "Due to academic pressure, students who were enrolled in all three sports were asked to withdraw from one of the three sports. After the withdrawal, the number of students enrolled in G was six less than the number of students enrolled in L, while the number of students enrolled in K went down by one.After the withdrawal, how many students were enrolled in both G and L?"

Hence, the answer is 6

Choice D is the correct answer.

 

Verbal Ability for CAT | CAT Reading Comprehension

Verbal Ability for CAT| CAT Para Jumble

Verbal Ability for CAT | CAT Sentence Elimination

Verbal Ability for CAT | CAT Paragraph Completion

Verbal Ability for CAT | CAT Critical Reasoning

Verbal Ability for CAT | CAT Word Usage

Verbal Ability for CAT| CAT Para Summary

Verbal Ability for CAT | CAT Text Completion

Verbal Ability for CAT | CAT Sentence Correction