CAT 2023 Question Paper | Quant Slot 3

CAT 2023 Quant was dominated by Arithmetic followed by Algebra. In Arithmetic, the questions were dominated by topics like Speed-time-distance, Mixture and Alligations. This year, there was a surprise. The questions from Geometry were relatively on the lower side as compared to the previous years. There were 8 TITA Qs this year. Overall this section was at a medium level of difficulty.

Question 18 : A rectangle with the largest possible area is drawn inside a semicircle of radius \(2 \mathrm{~cm}\). Then, the ratio of the lengths of the largest to the smallest side of this rectangle is

Video Explanation

Explanatory Answer

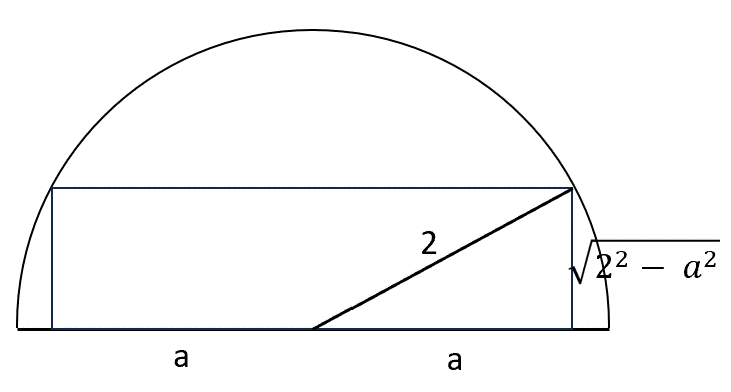
Let the length of the longer side (the side resting on the diameter) be equal to 2a.
Then the shorter side is given by \( \sqrt { 2 ^ { 2 } - a ^ { 2 } } \)
Area of the rectangle = \( A = 2 a \sqrt { 4 - a ^ { 2 } } \)
To maximize A, let us maximize A²
\( A ^ { 2 } = 4 a ^ { 2 } \left( 4 - a ^ { 2 } \right) \)
\( = 16 a ^ { 2 } - 4 a ^ { 4 } \)
\( = - 4 \left( a ^ { 4 } - 4 a ^ { 2 } \right) \)
\( = - 4 \left( a ^ { 4 } - 2 \times 2 \times a ^ { 2 } + 4 - 4 \right) = 16 - 4 \left( a ^ { 2 } - 2 \right) ^ { 2 } \)
The maximum value of \( A ^ { 2 } = 16 \) when \( a = \sqrt { 2 } \)
The shorter side will then be \( \sqrt { 4 - a ^ { 2 } } = \sqrt { 4 - 2 } = \sqrt { 2 } = a \)
Hence the ratio of longer to shorter side will be \( 2 a : a = 2 : 1 \)

The question is " A rectangle with the largest possible area is drawn inside a semicircle of radius \(2 \mathrm{~cm}\). Then, the ratio of the lengths of the largest to the smallest side of this rectangle is "

Hence, the answer is '\(2 : 1\)'

Choice A is the correct answer.