CAT 2020 Question Paper | DILR Slot 2

CAT Questions

The best questions to practice for CAT Exam are the actual CAT Question Papers. 2IIM offers you exactly that, in a student friendly format to take value from this. The questions in this Logical Reasoning set seen in CAT 2020 presents the idea of distributing beads in a grid, with a catch that the there is no one partiular arrangement. The idea of arrangements is taken one step further where we try to maximise the number of Red beads in any arrangement. We take the given conditions in and generate a powerful idea on how we can set a grid which helps us in solving other questions in the set as well. To check out about 1000 CAT Level questions with detailed video solutions for free, go here: CAT Question Bank

Twenty five coloured beads are to be arranged in a grid comprising of five rows and five columns. Each cell in the grid must contain exactly one bead. Each bead is coloured either Red, Blue or Green.

While arranging the beads along any of the five rows or along any of the five columns, the rules given below are to be followed:

1. Two adjacent beads along the same row or column are always of different colours.
2. There is at least one Green bead between any two Blue beads along the same row or column.
3. There is at least one Blue and at least one Green bead between any two Red beads along the same row or column.

Every unique, complete arrangement of twenty five beads is called a configuration.

Question 2 : What is the maximum possible number of Red beads that can appear in any conguration?

---

---

Video Explanation

---

---

Explanatory Answer

The idea here is to maximize the number of Reds in each row, this question will require us to draw the grid

Step 1: Try to draw as many Rs in a row as possible, since we know that 1R needs to have at least 1B and 1G between them, to maximize, we will need to have (ideally) exactly 1B and 1G
Move along the diagonal as you are trying to fill the Red beads since that way, we can also ensure that 2Rs do not come in contact in adjacent cells



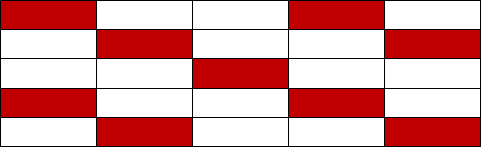
Step 2: Let's see if this is feasible with the given constraints. We will now need to fill in the G and B to see if it is feasible.



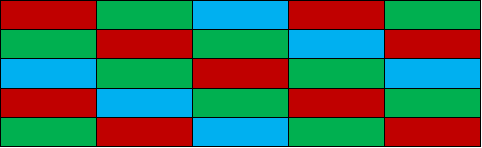
It is indeed feasible, by now you would have already noticed a pattern that moving along the diagonal (especially because of the non-repeating colours condition) is absolutely crucial to understanding the pattern in the grid. Here as well, you can see that the Bs and Gs are along the diagonal.

---

The question is "What is the maximum possible number of Red beads that can appear in any conguration?"

Hence, the answer is, " 9"

Verbal Ability for CAT | CAT Reading Comprehension

Verbal Ability for CAT| CAT Para Jumble

Verbal Ability for CAT | CAT Sentence Elimination

Verbal Ability for CAT | CAT Paragraph Completion

Verbal Ability for CAT | CAT Critical Reasoning

Verbal Ability for CAT | CAT Word Usage

Verbal Ability for CAT| CAT Para Summary

Verbal Ability for CAT | CAT Text Completion

Verbal Ability for CAT | CAT Sentence Correction